So we have two sets of solutions x = 1 2 π 2 k π or x = 3 6 1 1 π 2 k 3I = e i π /2 Thus i i = (e i π /2) i = e i 2 π /2 = eπ /2 Thus i i is a real number!Jan 23, 19 · What about the value of tan(π/2)?
Sin Theta Equals 0 General Solution Of The Equation Sin 8 0 Sin 8 0
π/2
π/2-The trigonometric R method is a method of rewriting a weighted sum of sines and cosines as a single instance of sine (or cosine) This allows for easier analysis in many cases, as a single instance of a basic trigonometric function is often easier to work with than multiple are The R method is most often used to find the extrema (maximum and minimum) of combinations of trigonometric§37 #5 Find the general solution of the differential equation y"y = tan(t),0



Compound Angles Proofs
X→(π 2)− sec(x) tan(x) Solution We know that this limit can be computed simplifying sec(x) tan(x) = 1 cos(x) cos(x) sin(x) = 1 sin(x) ⇒ L = 1 C We now try to compute this limit using L'Hˆopital's rule Indeterminate limit ∞ ∞ Example Evaluate L = lim x→(π 2)− sec(x) tan(x) Solution This is an indeterminate limitOct 15, 15 · The secant function can't be evaluated in pi/2 Just apply the definition and evaluate sec(x)=1/cos(x) Thus, sec(pi/2)=1/cos(pi/2) But since cos(pi/2) is zero, this division is not legit We can calculate the limit, so see if it's positive or negative infinite Since the cosine function is positive before pi/2 and negative after, we will have that lim_{x\\to (pi/2)^} sec(x)=infty, and lim_{xMassimiliano Feb 17, 15 The answer are x = 6 π 2 k π and x = 6 1 1 π 2 k π, x = 6 5 π 2 k π and x = 6 7 π 2 k
Mar 17, 16 · How do you prove #sin (π/2 – x) = sin (π/2 x)#?−π/2 = 4 2 Find the area inside the cardioid r = 1cos θ Answer The cardioid is sonamed because it is heartshaped Using radial stripes, the limits of integration are (inner) r from 0 to 1cos θ;Aug 28, · Inverse Trigonometric Formulas Trigonometry is a part of geometry, where we learn about the relationships between angles and sides of a rightangled triangleIn Class 11 and 12 Maths syllabus, you will come across a list of trigonometry formulas, based on the functions and ratios such as, sin, cos and tanSimilarly, we have learned about inverse trigonometry concepts also
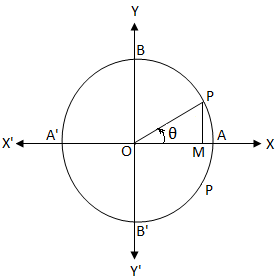
So 90° = π / 2 radians We usually suppress the unit of measurement "radians" since it is understood if no other units for angles is specified Also, since an angle in radians is defined as the ratio of two lengths, L/r, it is dimensionless Since 90° = π / 2 radians, to four significant figures, one radian equals 180°/ π = 5730°1 = π/2 Recall Polar coordinates in a plane Example Express in polar coordinates the integral I = Z 2 0 Z y 0 x dx dy Solution Recall x = r cos(θ), y = r sin(θ), θ 0 = π/4, θ 1 = π/2 The lower integration limit in r is r = 0 The upper integration limit is y = 2, that is, 2 = y = r sin(θ) Hence r = 2/ sin(θ) y 2 2 45 90 x 2 = y(outer) θ from 0 to 2π



Solved 3 Exprcss G Z Sin X Cos 2x In Terns Of X P 2 Chegg Com


Pdglive
Consider the following x = tan 2 (θ), y = sec(θ), −π/2 < θ < π/2 (a) Eliminate the parameter to find a Cartesian equation of the curveFind all solutions in the domain − 2 π, 2 π 2\pi,2\pi − 2 π, 2 π to the equation cos ( 4 x ) cos ( 2 x ) = 0 \cos(4x) \cos(2x) =0 cos ( 4 x ) cos ( 2 x ) = 0 We haveIn decimal form it is approximately 070 Here is a more algebraic way to see it De Moivre showed that e ix = cosx isinx so that e i π /2 = cos(π /2) isin(π /2) = i, for example


What Is The Exact Value Of Cot Pi 2 Socratic



G Pi 2 Physicsmemes
(4π2) t− 5 (4π2)2 By switching back to x we get y = 1 x2 (c 1 cosπlnx c 2 sinπlnx) 1 (4π 2) lnx− 5 (4π 2) 2 Plugging in the initial values gives c 1 − 5 (4π ) = 0,− 1 e c 1 1 (4π2) − 5 (4π2)2 = 0 which is not possible, so the boundary value problem has no solution 15 y00 λy = 0,y0(0) = 0,y(π) = 0 r2 λ = 0Oct 01, 17 · A pendulum is an object consisting of a mass suspended from a pivot so that it can swing freely The mathematics of pendulums are governed by the differential equation \frac{\mathrm{d}^{2}\theta}{\mathrm{d}t^{2}} \frac{g}{l}\sin\theta =Sin (π/2x) = cos x Now, we are aware of the expanded form of sum and difference of angle of cos Now, we will use the above concept for finding the values of sum and difference of angle of sin Sin(x y) can be written as cos π/2 –(x y) which is equivalent to cos (π/2 x)y Now, with the help of identity (2), we can write
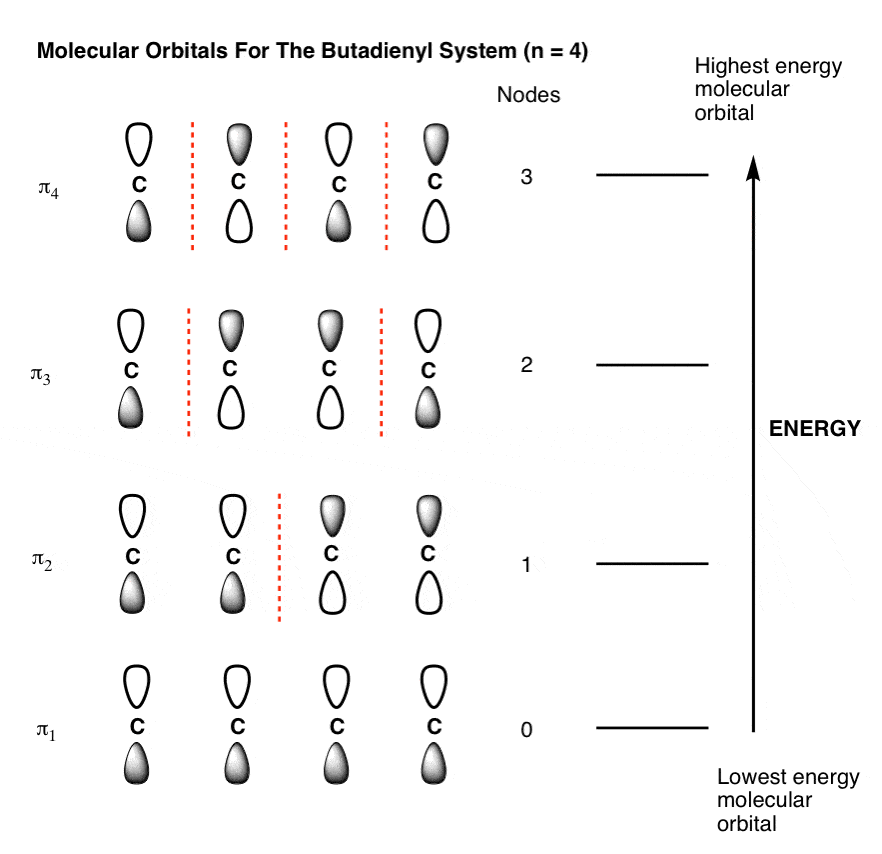


The Pi Molecular Orbitals Of Butadiene And How To Draw Them



Find Y Cosec Pi 2 X Y Cos X Cot Pi 2 X Sin Pi 2 X Maths Trigonometric Functions Meritnation Com
π 2 − 4 π X n≥1 cos(2n−1)x (2n−1)2 Since the series P 1 n2 converges, the MWeierstarss test implies that the above series converges In the above examples we have used the following identities, Z L −L g(x) dx = (2 RL 0 g(x) dx if g is even 0 if g is odd Using that the sum of two even functions is even and the sum of two oddNotice that as the angle gets larger and approaches π/2 rads, the opposite side gets larger and the adjacent side shrinks to 0 This means that tan(π/2) is equal to the expression 1/0 Division by 0 is undefined, so the function tan(π/2) is undefined and has no accepted valueThe trigonometric reduction formulas help us to "reduce" a trigonometric ratio to a ratio of an acute angleIf the acute angle is a common angle, this technique helps us to find the ratio For example, imagine you need to find cot 300° We can say that 300° =270°30° By the reduction formula for



Trig Arclength And Radians
.jpg)


Shifting Angle By P 2 P 3p 2 2p Finding Value Of Trignometric F
π/2 0 sin2k1 t dt = Z 1 0 (1−y2)k dy, we can use binomial expansion to show that the integral is equal to Xk r=0 k r (−1)r (2r 1) Then, the desired result follows by induction Choe's Proof (3)Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorClick here👆to get an answer to your question ️ x→pi/2 cotxcosx (pi2x)^3 equals


Solved Can Someone Explain How Sin X Pi 2 Becomes The Chegg Com



If 2 Sin X 1 Pi 2 X Pi And Sqrt2 Cos Y 1 3pi 2 Y 2pi F


