Sin 2 x = tan x (1 cos 2 x) \sin 2x=\tan x (1\cos 2x) sin 2 x = tan x (1 cos 2 x)Tan2x Formulas Tan2x Formula = 2 tan x 1 − t a n 2 x We know that tan (x) = sin (x)/cos (x) Then, tan2x formula = sin (2x)/cos (2x) Tan 2x can also be written in terms of sin x and cos x, Tan2x Formula in terms of cos x = 2 s i n ( x) c o s ( x) c o s 2 x − s i n 2 x You can check some important questions on trigonometry and trigonometry all formula from below 1 Find cos X and tan X if sin X = 2/3 2 In a given triangle LMN, with a right angle at M, LN MN = 30 cm and LM = 8 cm Calculate the values of sin L, cos L, and tan L 3

Sin2x Cos2x 1 Proof
Sin 2x tan 2x formula
Sin 2x tan 2x formula-The equation we want to solve is \sin(2x)\tan(x) You deduced correctly that we now have to solve 2\sin(x)\cos(x)\frac{\sin(x)}{\cos(x)}=0 which we can rewrite to 2Solve sin(2x) tan(x) = 0 Solving a Trigonometric Equation To solve a trigonometric equation, we first need to simplify it by applying any relevant trigonometric identities
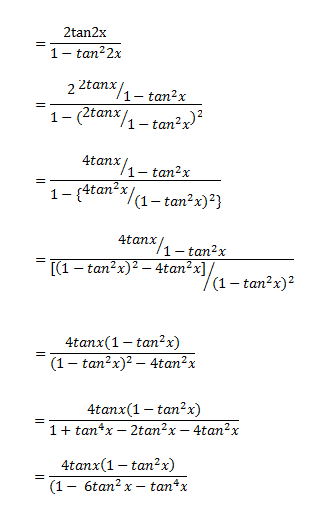



Trigonometry Functions Class Xi Exercise 3 3 Breath Math
Plot of the six trigonometric functions, the unit circle, and a line for the angle θ = 07 radiansThe points labelled 1, Sec(θ), Csc(θ) represent the length of the line segment from the origin to that point Sin(θ), Tan(θ), and 1 are the heights to the line starting from the xaxis, while Cos(θ), 1, and Cot(θ) are lengths along the xaxis starting from the origin #tan x=sinx/cos x, sin 2x = 2 sin x cos x and cos 2x = cos^2xsin^2x#, for the right hand side expression Explanation #2 tan x/(1tan^2x)=(2sin x/cos x)/(1(sin^2x/cos^2x)# Find sin 2x, cos 2x, and tan 2x from the given information tan x = −1/2 , cos x > 0 In trigonometry, the tangent halfangle formulas relate the tangent of one half of an angle to trigonometric functions of the entire angle They are as follows
Double Angle Formulas The trigonometric double angle formulas give a relationship between the basic trigonometric functions applied to twice an angle in terms of trigonometric functions of the angle itself Tips for remembering the following formulas We can substitute the values ( 2 x) (2x) (2x) into the sum formulas for sin \sin sin andFree trigonometric identities list trigonometric identities by request stepbystepSolve for x 2sin(2x)=tan(2x) Rewrite in terms of sines and cosines Substitute Take the inverse sine of both sides of the equation to extract from inside the sine The exact Divide each term in by Cancel the common factor of Tap for more steps Cancel the common factor Divide by Divide by The sine function is positive in the
cos(ax)cos(bx) = 1 2cos((a − b)x) 1 2cos((a b)x) These formulas may be derived from the sumofangle formulas for sine and cosine Example 726 Evaluating ∫ sin(ax)cos(bx)dx Evaluate ∫sin(5x)cos(3x)dx Solution Apply the identity sin(5x)cos(3x) = 1 2sin(2x) 1 2sin(8x)You can put this solution on YOUR website!Equation systems Step by Step;



Www Alamo Edu Contentassets 35e1aad11a064ee2ae161ba2ae3b2559 Analytic Math2412 Double Angle Power Reducing Half Angle Identities Pdf




The Value Of Lim X Pi 2 Tan 2x Sqrt 2sin 2x 3sinx 4 Sqrt Sin 2 X 6sinx 2 Is Equal To
\(\cos 2X = \frac{1\tan ^{2}X}{1\tan ^{2}X} Since, \tan X = \frac{\sin X}{\cos X} \) Hence, \(\cos 2X = \frac{1\tan ^{2}X}{1\tan ^{2}X} \) Solved Examples Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formula \(tan(ab) =\frac{ tan a tan b }{1 tan a tanb}\) So, for this let a = b , it becomes \(tan(aa) =\frac{ tan a tan a }{1 tan a tana}\) \(Tan 2a =\frac{2tan a}{1tan^{2}a} \) Practice Example for tan 2 theta QuestionSin(2x) = 2tan(x) /(1 tan 2 (x)) Sin 2x Formula in Terms of Cos Sin 2x formula can be written in the terms of Cos or cosine function only For this formula, we use the basic trigonometric identity of the relation between sin and cos and convert the sinx in the formula of sin2x in terms of cosx The formula for sin2x in terms of cos is Sin 2x = 2 sinx cosx




How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic



1
In Trigonometry Formulas, we will learn Basic Formulas sin, cos tan at 0, 30, 45, 60 degrees Pythagorean Identities Sign of sin, cos, tan in different quandrants Radians Negative angles (EvenOdd Identities) Value of sin, cos, tan repeats after 2π Shifting angle by π/2, π, 3π/2 (CoFunction Identities or Periodicity Identities) When trying to prove trig identities, it is often helpful to convert TAN functions into SIN/COS functions Proof Step 1 Start with the original equation to prove tan 2 x sin 2 x = (tan 2 x)(sin 2 x) Proof Step 2 Replace tan with sin/cos (sin 2 x/cos 2 x) sin 2 x = (sin 2 x/cos 2 x)(sin 2 x) Proof Step 3 Obtain a common denominator on left, simplify right (sin 2 x sin 2 x cos 2 x x=npi or x=npipi/4 where n is an integer As sinxtan^2x=sinx sinxtan^2xsinx=0 or sinx(tan^2x1)=0 ie either sinx=0=sin0 ie x=npi or tan^2x=1=tan^2(pi/4) ie tanx=tan(pi/4)or x=npipi/4 where n is an integer




Find Sin 2x Cos 2x And Tan 2x From The Given Chegg Com
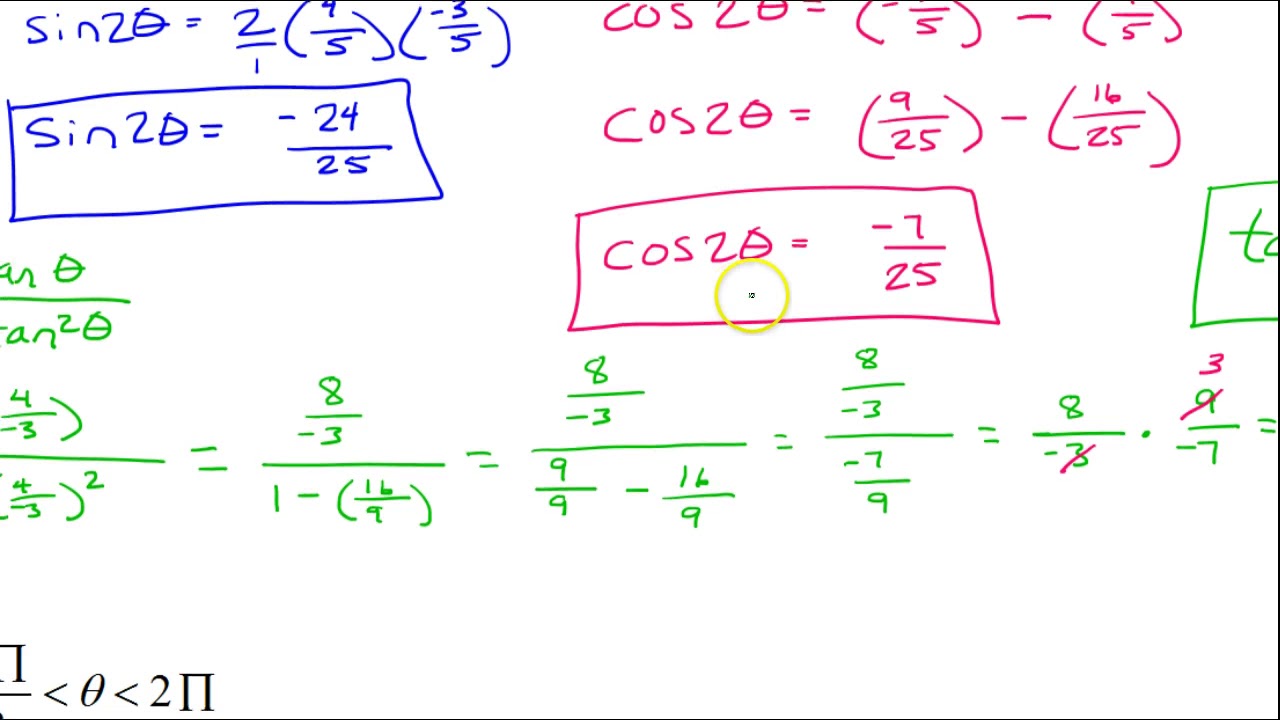



Day 3 Hw 1 To 2 Find Sin2x Cos2x And Tan2x Given One Trig Value And The Quadrant Youtube
Derivative Of sin^2x, sin^2(2x) – The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change with respect to a variable Common trigonometric functions include sin(x), cos(x) and tan(x) For example, the derivative of f(x) = sin(x) is represented as f ′(a) = cos(a) f ′(a) is the rate of changeThe equation of tangent at point (x 1 , y 1) with slope m is given by \(\rm (y y_1) = m (x x_1)\) \(\rm (y {\pi}) = 2 (x \dfrac{\pi}{2})\) y = 2x Hence, the equation of the tangent line to the curve y = 2x sin x at the point \(\left(\frac \pi 2, \pi\right)\) is 2x Download Question With Solution PDF ››We have to prove that (tan x)^2 (sin x)^2 = (tan x)^2 * (sin x)^2 Start from the left hand side (tan x)^2 (sin x)^2 use tan x = sin x / cos x
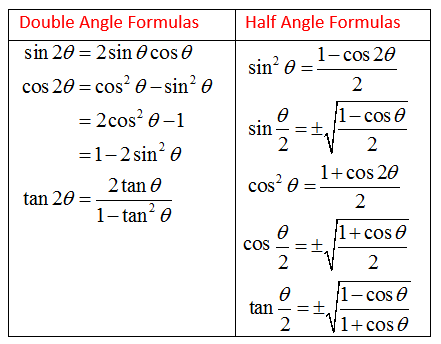



Double Angle Formula And Half Angle Formula Video Lessons Examples And Solutions
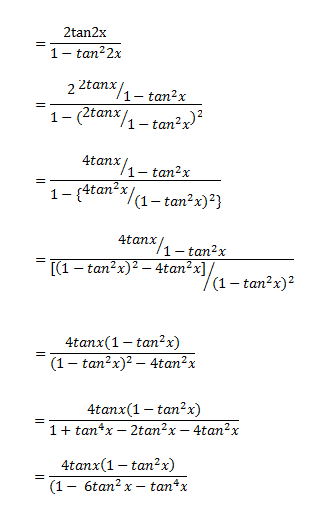



Trigonometry Functions Class Xi Exercise 3 3 Breath Math
FORMULAS TO KNOW Some trig identities sin2xcos2x = 1 tan2x1 = sec2x sin 2x = 2 sin x cos x cos 2x = 2 cos2x 1 tan x = sin x cos x sec x = 1 cos x cot x = cos x sin x csc x = 1 sin x Some integration formulas R xn dx = xn1 n1 C R 1 x dx = lnjxjC R ex dx = ex C R sin x dx = cos x C R cos x dx = sin xC R sec2 x dx = tan xC R tan x secOther calculators Integral Step by Step;Identities related to sin 2x, cos2x, tan 2x, sin3x, cos3x, and tan3x Sin 2x = Sin 2x = sin(2x)=2sin(x) cos(x) Sin(2x) = 2 * sin(x)cos(x) Proof To express Sine, the formula of "Angle Addition" can be used
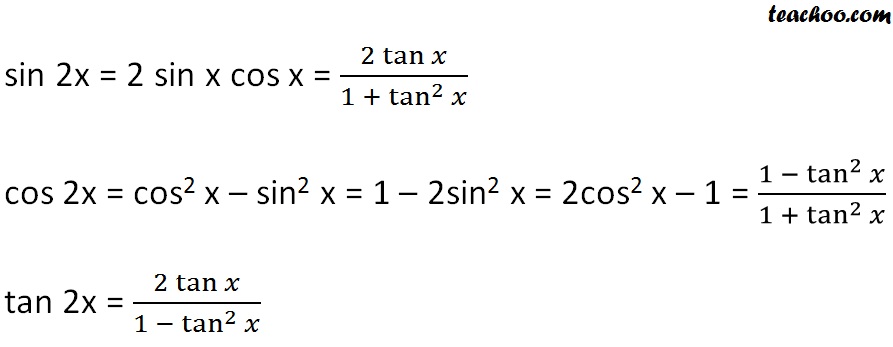



Double Angle Formulas Trigonometry Teachoo 2x 3x Formula Provi



Q Tbn And9gcswqgrwxfu3ry9lhuwrnhzq Lnjumaniii4kgzg4 Wagpp Cjba Usqp Cau


